Charting The Course: A Complete Information To Changing Fractions To Decimals

Charting the Course: A Complete Information to Changing Fractions to Decimals

Associated Articles: Charting the Course: A Complete Information to Changing Fractions to Decimals

Introduction

On this auspicious event, we’re delighted to delve into the intriguing subject associated to Charting the Course: A Complete Information to Changing Fractions to Decimals. Let’s weave attention-grabbing data and provide contemporary views to the readers.

Charting the Course: A Complete Information to Changing Fractions to Decimals

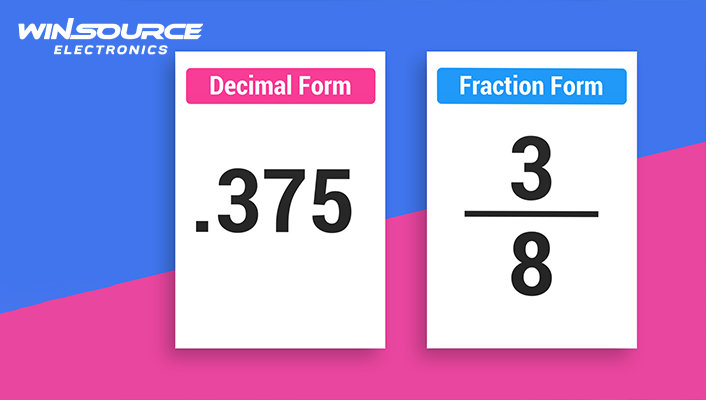
Fractions and decimals are two basic representations of numbers, each essential for understanding and manipulating numerical information. Whereas seemingly distinct, they’re intrinsically linked, representing the identical underlying worth expressed in numerous types. The flexibility to seamlessly convert between fractions and decimals is crucial for a variety of functions, from fundamental arithmetic and on a regular basis calculations to superior scientific computations and monetary modeling. This text gives a complete information to changing fractions to decimals, using varied strategies and illustrating the method with charts and examples. We are going to discover various kinds of fractions and tackle widespread challenges encountered through the conversion course of.

Understanding Fractions and Decimals:

Earlier than diving into the conversion strategies, let’s revisit the basic ideas of fractions and decimals.

• Fractions: A fraction represents part of an entire. It is expressed as a ratio of two integers, the numerator (high quantity) and the denominator (backside quantity). The denominator signifies the full variety of equal elements the entire is split into, whereas the numerator specifies what number of of these elements are being thought of. For instance, 3/4 represents three out of 4 equal elements.

• Decimals: A decimal is a illustration of a quantity utilizing a base-ten system. The digits to the fitting of the decimal level symbolize fractions with denominators which can be powers of ten (10, 100, 1000, and so forth.). For example, 0.75 represents 75/100.

Strategies for Changing Fractions to Decimals:

The core precept behind changing a fraction to a decimal is to divide the numerator by the denominator. Nonetheless, the complexity of this course of is dependent upon the character of the fraction.

1. Easy Fractions with Denominators which can be Powers of 10:

These are the simplest fractions to transform. If the denominator is 10, 100, 1000, or some other energy of 10, merely write the numerator and place the decimal level in response to the denominator’s worth.

Fraction	Denominator	Decimal Equal	Rationalization
7/10	10	0.7	Place the decimal level one place to the left of the numerator (7).
35/100	100	0.35	Place the decimal level two locations to the left of the numerator (35).
125/1000	1000	0.125	Place the decimal level three locations to the left of the numerator (125).
2345/10000	10000	0.2345	Place the decimal level 4 locations to the left of the numerator (2345).

2. Easy Fractions with Denominators that aren’t Powers of 10:

For fractions with denominators that aren’t powers of 10, we carry out lengthy division.

Instance: Convert 3/4 to a decimal.

Divide the numerator (3) by the denominator (4):

3 ÷ 4 = 0.75

Due to this fact, 3/4 = 0.75

Chart illustrating the lengthy division course of:

Step	Dividend	Divisor	Quotient	The rest
1	3	4	0	3
2	30	4	7	2
3	20	4	5	0

3. Combined Numbers:

Combined numbers consist of an entire quantity and a fraction (e.g., 2 1/2). To transform a blended quantity to a decimal, first convert the fraction half to a decimal utilizing the strategies described above, then add the entire quantity.

Instance: Convert 2 1/2 to a decimal.

1/2 = 1 ÷ 2 = 0.5

2 1/2 = 2 + 0.5 = 2.5

4. Improper Fractions:

An improper fraction has a numerator better than or equal to the denominator (e.g., 7/4). Convert it to a blended quantity first, then observe the tactic for blended numbers.

Instance: Convert 7/4 to a decimal.

7/4 = 1 3/4

3/4 = 0.75

1 3/4 = 1 + 0.75 = 1.75

5. Repeating and Terminating Decimals:

When changing fractions to decimals, you could encounter two varieties of decimals:

• Terminating Decimals: These decimals have a finite variety of digits after the decimal level (e.g., 0.75, 0.125). These sometimes end result from fractions the place the denominator has solely 2 and/or 5 as prime elements.

• Repeating Decimals: These decimals have a sequence of digits that repeat infinitely (e.g., 1/3 = 0.333…, 1/7 = 0.142857142857…). These are indicated by putting a bar over the repeating sequence (e.g., 0.3̅, 0.142857̅). Repeating decimals end result from fractions the place the denominator has prime elements aside from 2 and 5.

Chart illustrating Terminating and Repeating Decimals:

Fraction	Decimal Equal	Sort
1/2	0.5	Terminating
3/4	0.75	Terminating
1/3	0.3̅	Repeating
1/6	0.16̅	Repeating
1/7	0.142857̅	Repeating
5/8	0.625	Terminating
2/9	0.2̅	Repeating

Challenges and Issues:

• Lengthy Division: For fractions with bigger numbers, lengthy division will be time-consuming. Calculators can considerably expedite this course of.

• Rounding: When coping with repeating decimals, it is usually essential to around the decimal to a selected variety of decimal locations for sensible functions. The rounding methodology used (e.g., rounding up, rounding down, rounding to the closest digit) ought to be clearly acknowledged.

• Accuracy: The accuracy of the decimal illustration is dependent upon the variety of decimal locations used. Extra decimal locations present a extra correct illustration however may make the quantity much less manageable.

• Utilizing Calculators: Whereas calculators are useful, it is essential to know the underlying ideas of fraction-to-decimal conversion. Calculators can be utilized to confirm outcomes and deal with advanced calculations, however they should not exchange a basic understanding of the method.

Purposes of Fraction-to-Decimal Conversion:

The flexibility to transform fractions to decimals is important in quite a few fields:

• Finance: Calculating rates of interest, reductions, and revenue margins usually includes working with each fractions and decimals.

• Science: Many scientific measurements and calculations require using decimals, even when the preliminary information is introduced as fractions.

• Engineering: Exact calculations in engineering usually demand conversions between fractions and decimals to make sure accuracy.

• Cooking and Baking: Recipes continuously use fractions, however understanding the decimal equivalents is useful for adjusting recipes or utilizing measuring instruments.

• Knowledge Evaluation: Changing fractions to decimals facilitates information evaluation and statistical calculations, particularly when utilizing laptop software program or spreadsheets.

Conclusion:

Changing fractions to decimals is a basic mathematical talent with broad functions throughout varied disciplines. Whereas the method can vary from easy to extra advanced, mastering completely different strategies, together with lengthy division, and understanding the ideas of terminating and repeating decimals are important for correct and environment friendly calculations. This text has offered an in depth information, using charts and examples for example the completely different strategies and spotlight widespread challenges. By understanding these ideas, people can confidently navigate the conversion course of and apply this information to a variety of sensible conditions. The flexibility to seamlessly transfer between fractional and decimal representations enhances mathematical fluency and opens doorways to a deeper understanding of numerical ideas.

Closure

Thus, we hope this text has offered worthwhile insights into Charting the Course: A Complete Information to Changing Fractions to Decimals. We hope you discover this text informative and helpful. See you in our subsequent article!